
However, this test requires some assumptions so let's look into those first. We'll answer just that by running a paired samples t-test on each pair of exams.
SoĪre the sample means different enough to draw this conclusion?

However, very different sample means are unlikely and thus suggest that the population means aren't equal after all. So even if the population means are really equal, our sample means may differ a bit. We only have a sample of N = 19 students and sample outcomes tend to differ from population outcomes. Now, we don't have data on the entire student population. Generally, the null hypothesis for a paired samples t-test is that They hold the number of correct answers for each student on all 3 exams. Their data -partly shown below- are in compare-exams.sav. He needs to know if they're equally difficult so he asks his students to complete all 3 exams in random order. In order to have a more accurate view, we should a have a bigger sample, to make a more precise statement.SPSS Paired Samples T-Test Tutorial report this ad By Ruben Geert van den Berg under Statistics A-Z & T-TestsĪ paired samples t-test examines if 2 variablesĪre likely to have equal population means.Ī teacher developed 3 exams for the same course.Both the parametric and non-parametric approach don't provide enough evidence to support the claim the population mean volume for premix A is significantly greater than the mean volume for premix B.This means that we don’t have enough evidence to claim that the data is not normal. This means that we fail to reject the null hypothesis of normality, since the significance is greater than 0.05. Finally, we go to Plots, and we click at "Normality Plots". Then we select the variables "A" and "B". We go to "Analyze", "Descriptive Statistics" and "Explore". We are going to test the data for normality. In other words, we again don’t have enough evidence to support the claim that the population mean volume for premix A is significantly greater than the mean volume for premix B, in this case using a non-parametric approach. The significance of the test is \(p = 0.129\), which means that the difference is not significant (same as predicted before). We go to "Analyze", "Non-Parametric tests", "2 related samples", and then we click at "Wilcoxon". Since the statistics is not less than or equal to the critical value, we fail to reject the null hypothesis. The \(x\) statistics corresponds to the number of the less frequent sign. We need to compute the table with the differences represented with a "+" or "-". We use nonparametric analysis when either the populations are not normally distributed, or if we want to make a claim about the median of premix A and B. The significance of the test is \(p = 0.141\), which means that the difference is not significant. Then we select the variables "A" and "B" and we click OK.
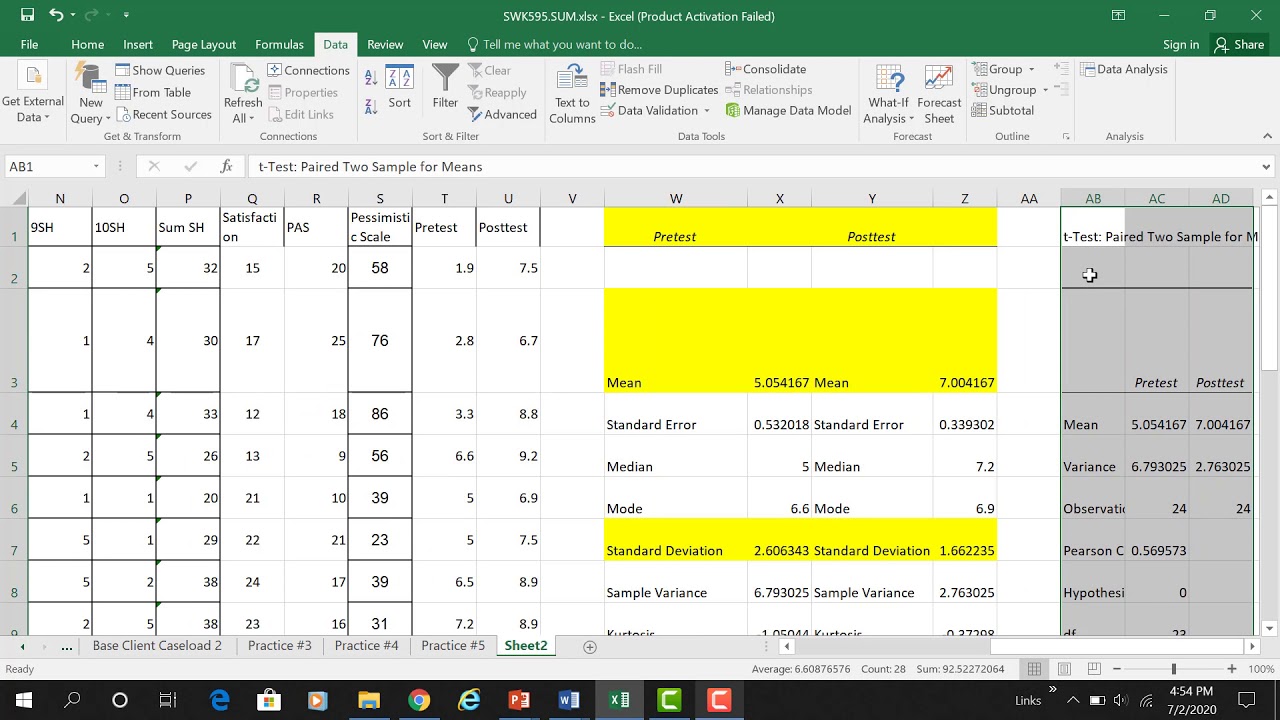
Then we go to "Analyze", "Compare Means" and "Paired samples T-test. Since the t-statistics is less than the critical value, we fail to reject the null hypothesis, which means that we don’t have enough evidence to support the claim that the population mean volume for premix A is significantly greater than the mean volume for premix B. & =1.746075\]įor 5 degrees of freedom, and a significance level of \(\alpha =0.05\), the critical t-value is equal to The claim made in the patent is translated into In this case our aim is to make a statement about the population mean volumes for premix A and B. There are several ways we can determine whether the volumes differ significantly. In the patent process, it was claimed that a significantly greater volume was obtained by premix B. Where the data represents the volume of cakes obtained by using 6 different recipes and two different premixes A and B.


 0 kommentar(er)
0 kommentar(er)
